What Is Perspective?
 |
|
|
Simple one-point perspective drawing |
|
|
|
|
|
|
The Basics of Perspective
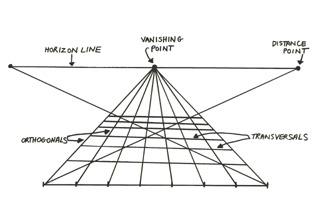
Linear perspective is a mathematical system for projecting the three-dimensional world onto a two-dimensional surface, such as paper or canvas. In brief, this type of perspective begins with a horizon line, which defines the farthest distance of the background and a central vanishing point. To this vanishing point, orthogonals may be drawn from the bottom of the picture plane, which defines the foreground of the space. The orthogonals, vanishing point, and horizon line establish the space in which the artist may arrange figures, objects, or architecture such that they appear to exist in three dimensions. Once these basic elements have been set in place, the artist may add further elements to create a more complicated, yet more realistic, space. For example, to represent a square-tiled floor, the artist chooses another point on the horizon line, called the distance point, and draws a line through the orthogonals to a point at the bottom of the picture plane. The points at which this line bisects the orthogonals establish the points at which horizontal lines, called transversals, may be placed. These lines represent the perspectively correct regression of the square tiles into space (see diagram). These elements of linear perspective link the science of three-dimensional geometry with the art of illusionistic representation.
Art-Historical Meanings of Perspective
In the 13th and 14th centuries, before linear perspective was discovered, artists occasionally employed something called reverse perspective, in which parallel lines splay rather than converge as they approach the horizon line. One of the rules set forth in an early artists' manual is that elements above the eye of the viewer tend downward (like roofs), while elements below the viewer’s eye tend upward (like tables). While arbitrary tilting of lines upward and downward can create unusual effects, this is generally considered to be a significant step in the progression toward the rational application of linear perspective.
It was not until the Renaissance that artists began to refine this science. Linear perspective soon emerged as the tool for artists to capture the world around them in a remarkably illusionistic manner (this was the same time that cartographers were mapping the surface of the earth using a similar system of mathematical projection). Masaccio's (1401–28) Trinity (1427–28), considered to be the first accurately perspectival painting in the Western tradition, introduced the relationship between linear perspective and subject matter in art. The painting is divided into three levels: the figure of God stands on a tomb above and behind Christ crucified on the cross; the Virgin Mary and St. John the Baptist stand at the base of the cross; and two donors, who commissioned the painting, kneel on either side of the cross at the lowest level. The figures are harmoniously organized underneath a barrel-vaulted ceiling. The figure of Christ and the barrel vault are rendered as if seen from below. The figures of Mary and John are rendered again as if from below, but just lower than Christ. The two donors, given the lowest vanishing point, are rendered as if seen, directly in front of the viewer’s eye. In this way, the painting provides the illusion that the viewer is looking at sculptural forms that exist in three dimensions and rise vertically in space. Yet the accurate, illusionistic representation of space in this work has no obvious theological meaning. It has much more to do with the artist exhibiting his skill—injecting his own thoughts into this religious image.
Unusual Kinds of Perspective
Some artists of the Renaissance were not as concerned with putting their subjects in perfect perspective as they were with making religious statements. For example, in pictures that include the Virgin Mary the vanishing point is often intentionally placed on Mary's womb to indicate her place as the mother of Christ. This placement of the vanishing point has religious significance and may not be related to the intention to create a rational perspectival space. Further, if the artist chooses a short distance between the distance point and the vanishing point then the perspective will appear warped. The distance between the distance point and the vanishing point should, in theory, correspond exactly to the distance between the viewer’s eye and the picture plane. When the distance between the points is small, the viewer must place his or her eye at this same distance from the painting directly in front of the distance point in order to see the work with no distortion whatsoever. When the viewer stands back from the work, the space in the image will appear distorted.
Anamorphosis (from the Greek, “something without form”) involves stretching an ordinary linear-perspective image in one or more directions to obscure its original form. To achieve this, the artist draws a grid over the original image and then translates the image point by point to a grid that has been stretched. If the viewer looks at the image directly, it appears formless and amorphous. In order to recognize the image, the eye of the viewer must be positioned from a particular spot, generally off to the side, and from this point the image appears in linear perspective.
Curvilinear perspective is an alternate to linear perspective. Although technically all straight lines are curved, curvilinear lines are suppressed in Western painting—that is, straight lines are represented as straight rather than arced. In the 19th century, a group of artists made an attempt to return curvilinear perspective to painting, but the idea was short lived because it presented a philosophical problem. When observing lines in the real world, such those of as walls and buildings, the lines appear curved. (Think of standing in front of a long wall, and looking left and right: The top of the wall seems to curve up from either side.) It follows that a wall in a painting, drawn with straight lines, can also seem curved. Therefore, if those curves are represented in painting they will seem doubly curved. This tension between reality and the representation of reality in painting posed a challenge to the painters who employed this technique.
Perspective and Nature
There are some elements of representation over which linear perspective has no power, such as landscapes, faces, and organic forms, for this type of perspective only describes linear things. In his Landscape with Saint John on Patmos (1640), Nicolas Poussin (1593/4–1665) used linear perspective to demonstrate his knowledge of geometry. In the ruins in the foreground, a cube, a three-dimensional rectangle, and a cylinder are represented in perfect perspectival form, each illustrating a different aspect of foreshortening. Yet the landscape in which the objects and the figure of Saint John exist does not adhere to the rules of linear perspective. The pathway recedes into the distance giving an illusion of depth, but nature twists and turns and rises and falls in its unpredictable, organic way.
Modern Art and Linear Perspective
Since the Renaissance, painters have reworked and refined linear perspective. The American 19th-century realist Thomas Eakins (1844–1916) created remarkably accurate outdoor scenes, with shadows painted so precisely that art historians have been able to determine, based on their knowledge of where the works were painted, the exact date and time of day he painted them. Some critics have argued that perspective was destroyed by modern artists such as Pablo Picasso in the early-20th century. In works such as his portrait of Daniel-Henry Kahnweiler (1910), Picasso sought to break up the picture plane and divide the forms into individual geometric pieces. Yet early modern artists did not actually overthrow perspective; they borrowed from it, elaborated upon it, and redefined it for the viewer. That linear perspective is still very much a part of representation today is evident in video games, which employ the most exact perspective in the Western tradition. In computer software, all figures and objects are drawn using a perfect geometric grid. Regardless of the vantage point from which the player views figures in a game, all figures, objects, and elements in the settings adhere to the established rules of representation. Perspective is both an exacting art and an exacting science that is still very much all around us.
Adapted from a lecture titled “What Is Perspective?” by Jim Elkins. www.jameselkins.com.